
Botany online 1996-2004. No further update, only historical document of botanical science!
Pigmentation patterns of shells of mollusc - a natural picture book to study dynamic systems
Elementary pattern on the sea shells: traces of a stable pattern, oscillations and travelling waves
Arrangement of leaves and staggered dots on shells - two corresponding patterns
Of course, the structure of a higher organism is more complex than can be achieved by the interpretation two orthogonal gradients. The generation of sub-structures like legs, wings or eyes, requires secondary pattern forming reactions. They must be initiated at precise position and the resulting pattern must obtain the correct orientation with the established axes of the embryo. Secondary fields have been proposed to be formed around the intersection of two determination borders (Meinhardt, 1983a,b). Let us first regard two neighbouring cell types, A and P and assume that both co-operate to produce a new substance m. For instance, the P cells may produce a necessary cofactor while only the A cells are able to generate the final product. In this way, the m production is restricted to the common border. If diffusion is involved, its concentration provides a measure for the distance from the border. It is therefore appropriate for the internal organization of the A and the P region. Although the pattern is symmetric in respect to the border, the resulting pattern can be asymmetric since the A and P cells can respond differently. In the vertebrate limb an extreme case is realized: only the A cells respond at all.
A border that separates two cell types along the anteroposterior axis surrounds an embryo in a belt-like fashion. To determine the position of a limb along this line a co-operation between a second pair of cell types is required. Their border must be oriented perpendicular to the first. According to this view, secondary fields are generated around the intersection of two borders. Therefore, secondary fields consist from the beginning of four different quadrants (or, as in the insect leg, of at least three sectors). Simplifying an early embryo as a cylinder, for any reasonable subdivision along the anteroposterior and the dorsoventral axis these intersections occur in pairs, one at the right and the other at the left side of the embryo. They have opposite handedness, a feature crucial for the formation of legs, wings, eyes, etc. In contrast to the classical model, the secondary field is assumed to be never without an internal structure since the formation of the borders is the primary event. Many classical observations of insect and vertebrate appendages become explicable (Meinhardt, 1983a, 1983b). This model has found much support by recent investigation on the molecular-genetic level (Vincent and Lawrence, 1994; Martin, 1995, Meinhardt, 1994). The engrailed/wingless border mentioned above involved in the generation of segmentation is also a the primary border for the generation of insect legs and wings.
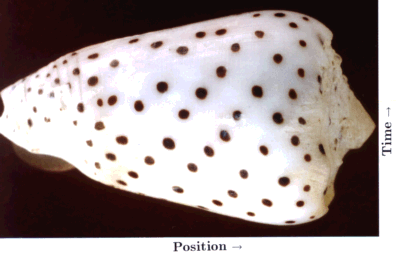
A special case of biological pattern formation is the emergence of the pigment patterns on the shells of molluscs. These patterns are of great diversity and frequently of great beauty. The shells consist of calcified material. The animals can increase the size of their shells only by accretion of new material along a marginal zone, the growing edge of the shell. In most species, pigment becomes incorporated during growth at the edge. In these case, the pattern formation proceeds in a strictly linear manner. The second dimension is a protocol of what happens as function of time. The shell is, so to say, a space-time plot. The shells provide a unique situation in that the complete history of a highly dynamic process is preserved.
In normal development, a strong evolutionary pressure exists to reproduce faithfully a given structure. In contrast, the functional significance of the pigment patterns on shells is not clear. Many molluscs live buried in the ground and some are covered with an opaque layer, the periostracum. Thus, there is presumably no strong selective pressure on the shell patterns. Together with Martin Klingler I have shown that the same types of interactions we have proposed for pattern formation during embryonic development are also able to account for shell patterning (Meinhardt and Klingler, 1987). Very different appearing shell patterns can be reproduced by small variation of the parameters or minor changes in the underlying mechanism. An extensive treatment of models for the elementary and the more complex patterns is provided in a recent book (Meinhardt, 1997, 1998). The book is accompanied by a floppy disk that allows a reproduction of the simulations on a PC and includes the source code. It contains also an integration of these models into a three-dimensional description of the shell shape by Fowler and Prusinkiewicz.
Basic elements of the shell patterns are lines parallel, perpendicular or oblique to the direction of growth. Keeping their space-time character in mind, lines parallel to the direction of growth (and thus usually perpendicular to the growing edge) indicate that pigment production occurs at particular positions that are separated by regions without pigment production. This is the usual situation as discussed above for normal development. The formation of a particular structure is restricted to a particular position. As shown above, it requires that the self-enhancing reaction is more or less locally confined while the antagonistic effect has a long range. In contrast, pigmented lines perpendicular to the direction of growth (usually parallel to the growing edge) are traces of a more or less synchronous oscillation in pigment production. In terms of the model, this occurs if the antagonistic reaction follows the self-enhancing reaction too slowly. The activation increases in an avalanche-like manner. It is only somewhat later that the accumulating inhibitor (or the removal of all the substrate) causes a collapse in the activator production. A refractory phase follows. A new activation can be triggered only after the decay of the remaining inhibitor. In other words, a longer time constant of the antagonistic reaction can lead to oscillations. A rapid diffusion of either the activator or the inhibitor can lead to their synchronization.
Lines oblique to the growing edge result from travelling waves of pigment production. In the model, they result if the activator has a small diffusion range while the antagonistic substance is nearly non-diffusive. An activated region can "infect" its neighbouring region such that, after a certain lag phase, it becomes fully activated too. Such a cell will infect a neighbouring region, and so on. The situation is very similar to the wave-like spread of an epidemic. One person can infect its neighbours. The full development of a sickness is also based on a self-enhancing effect, the replication of the virus. Some time after bursting virus proliferation, the immune system begins to acts antagonistically. It captures the virus and the person will become healthy again. For the spread of the epidemic it is crucial that only the virus, but not the immune response is transmitted from one individual to the next.
In the following, only a single pattern will be discussed: dots that are arranged along oblique lines. The simulation shown in Fig. 19c is based on an interaction of one activator and two inhibitors that act in an additive way. One inhibitor has a long range (red) and a short time constant, the other a short range but a long time constant (green). The activated regions are shown in black. The first inhibitor causes the separation of the activations along the space-, the other along the time co-ordinate. In this case, the poisoning of the maximum leads to their disappearance and to their new trigger at a displaced position.


Fig. 19: Staggered dots on shells (a) and the helical arrangement of seeds on a fire cone (b) are proposed to be based on an analogous mechanism. A corresponding pattern is produced in a linear array of cells as function of time by the interaction of one activator (black) and two antagonists. The resulting pattern consists of patchy signals arrangend on oblique rows. Calculated on a ring (cut open) and plotted as function of time (upwards)

Fig. 20: Simulation as in Fig. 19c. On a smaller ring, only a single spiral is possible. The angular displacement corresponds to the golden angle (see Meinhardt et al., 1998)
The regular initiation of leaves behind the tip of a growing shoot, called phyllotaxis, seems to have nothing in common with any pattern on sea shells, but this impression is misleading. As in shells, also on a growing shoot the new pattern elements appear in a narrow zone in the course of time. The tip of the shoot, the so-called meristem, consists of undifferentiated, rapidly dividing cells. Only cells just leaving this zone are able to form new leaves. According to classical models, the initiation of a new leaf is inhibited by existing leaves (Schoute, 1913). Therefore, a new leaf can be initiated only at a certain distance from the last formed leaf. In this way, a certain distance is maintained between the sites of leaf initiation.
In many plants, leaves are initiated along spirals. Seeds on fire cones have a corresponding arrangement (Fig. 19b). Such patterns result if not only the last, but also the next-to-last leaf has a repelling influence on initiation of a new leaf. This can be simulated with the basic model. However, since the inhibitor has to diffuse rapidly, the directing cue resulting from the penultimate leaf on the positioning of a new leaf is minute. Therefore, such a mechanism is not robust against small perturbations, in contrast to the observations. In the simulations, there is a tendency to fall back to an alternating (distichous) or pair-wise, 90°-rotated (decussate) arrangement. Helical patterns can emerge very reliably if, as in the shifted dot model discussed above, two separate inhibitions are assumed (Fig. 19-21; Meinhardt et al., 1998).



Fig. 21: Formation of helical pattern formation in the circular leaf forming zone
Fig. 22: Formation of distichous pattern formation in the circular leaf forming zone
Fig. 23: Formation of decussate pattern formation in the circular leaf forming zone
According to this model, the helical arrangement does not result from the inhibitory influence of the earlier leaves, but the from the long-lasting memory of cells in the leaf forming zone that a leaf has been formed at this position. Since this memory is based on a nearly non-diffusing substance, it remains localized. Fig. 21 shows the simulation around a ring of cells, the leaf forming zone, as function of time. Initiation of new signals occur with a displacement close to the golden angle (137,5°). Fig. 22. shows a the same simulation at the leaf-forming zone. The periodic formation of the signal, each at a shifted position, is clearly visible. Depending on the range of the inhibition and the diameter of the ring at the leaf forming zone, periodic generation of signals with a distichous (180° alternating, Fig. 22) or with a decussate (successive opposite pairs emerging with at 90° to each other, Fig. 23).